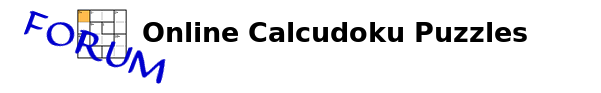Segmentation. (My purpose in this topic is to summarize conclusions previously obtained in topics like: “Why in a 4x4 numbers in corners are those in inner area (1)”, “Why in a 4x4 numbers in corners are those in inner area (2)”, “Something I’ve always been curious about” and “The “rule of quadrants” - 6x6 difficult from 09-May-2012”.
I define a
“segmentation” as the division of the full puzzle in various “elements”: cells, rectangles, or squares.
We can colour the elements so assigning them to an specific “colour”. We say that we have a
“correct segmentation” when it is possible, by transposition of lines, to group the elements corresponding to two of those different colours in such a way that we obtain two “complementary squares”, which sides are p and q (p + q = r, being r the range, or size, of the puzzle). If we have a “correct segmentation” we can apply the formulas and conclusions exposed here. In this case, tough apparently distributed randomly over the puzzle, those “elements” form “virtual squares” because they can be grouped.
What is happening here (Graphic 1)?. Why the numbers contained in the blue elements are the same as those in the yellow square?.
We have the same situation here (Graphic 2):

This occurs because the squares created by transposition of lines have the same side. But if the side of the squares is different we arrive to situations like this:
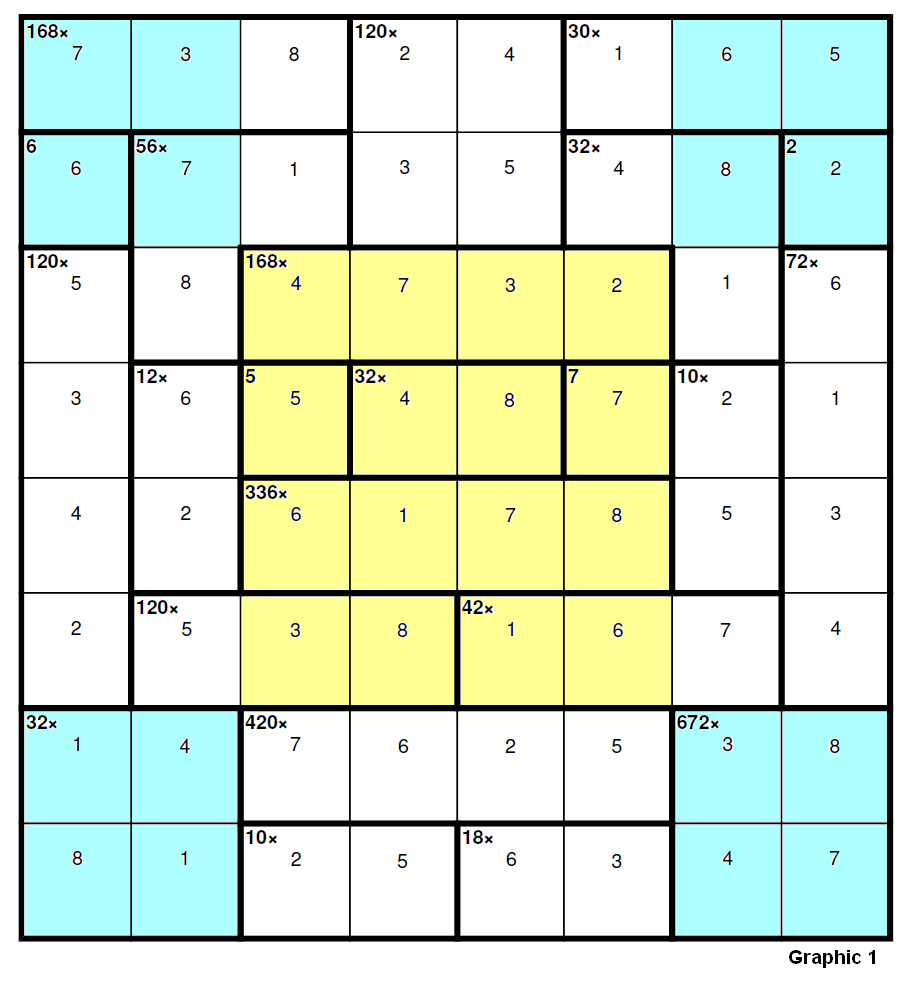
In this case (Graphic 3), the violet square contains
exactly the same numbers of the blue square plus a “full set” of 1, 2, … , 9, that is, a complete line (numbers in dark violet for a better identification).
Or here (Graphic 4), where the yellow square contains the numbers in the blue square, identified in dark yellow, plus two complete lines, that is, twice the full set 1, 2, … , 6:

And here (Graphic 5), where the green elements include the numbers in the brown square (identified as dark green) plus two complete lines, that is, twice the full set 1, 2, … , 6:

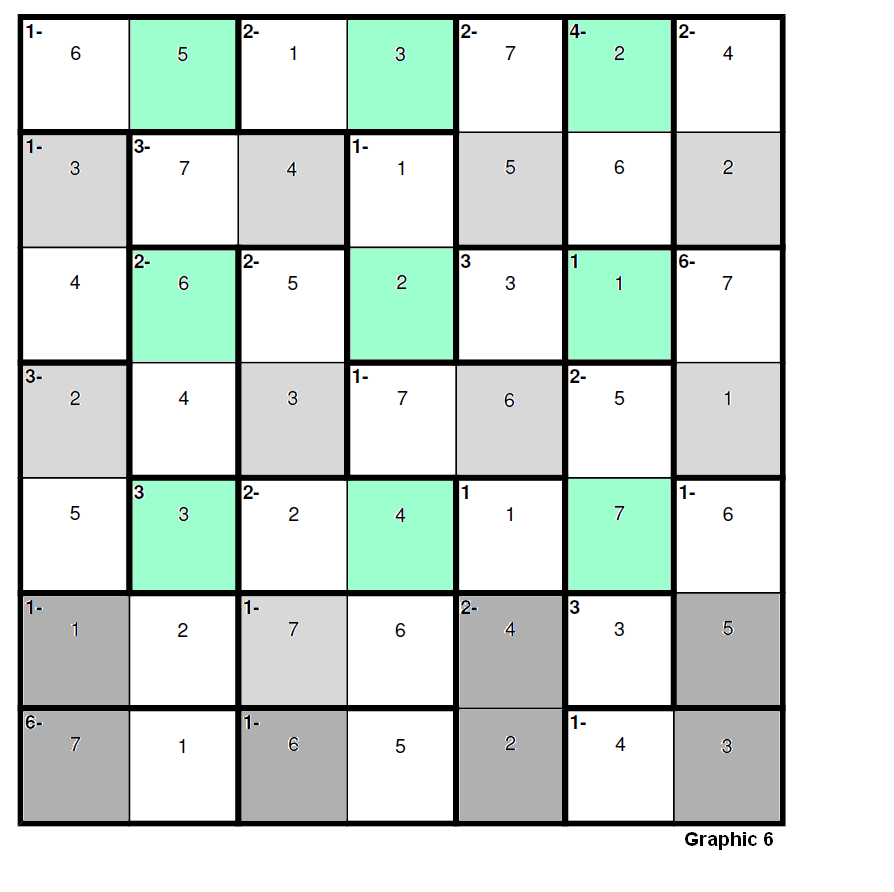
Finally, the last example: This 7x7 (Graphic 6), where we can group, transposing lines, the grey and green “elements”, in this case cells, to form two “complementary” squares, the gray square would contain all numbers in green plus one line, the full set 1, 2, … , 7 (in dark gray).

The demonstration of such a behaviour:
The main equation:
S2 = S3 + (q – p) . L Moving physically the lines (and the adhered colours), transposing them, does not violate the “calcudoku-sudoku” main rule (any line must contain 1, 2, … , r). Inversely, we could leave the numbers in their places but “move” the colours, with the same result. The interesting thing is that when the relationship between the numbers in the “virtual squares” is known, the rules of the addition, the multiplication and the parity could perfectly be applied.
Now, for instance, we can explain why the corners in a 4x4 must be the same numbers as those in the inner area: we are dealing with a “correct segmentation” of the 4x4 (topics “Why in a 4x4 numbers in corners … ").
And also we can see why the inner area (I) of any puzzle has a sum which is equal to the sum of the corners, C, plus (r - 4) times the value of a line: Since we have a “correct segmentation”, the inner area contains the corners plus r – 4 sets of 1, 2, … , r, and this is because the inner area has a side of r - 2 and the other “virtual” square (the corners) has a side of 2 then q – p = r - 2 - 2 = r – 4 (topic “Why in a 4x4 numbers in corners are those in inner area (2)”).
In the above demonstration the result
S1 = S4 (topic “Something I’ve always been curious about” and also the jotempe’s post “The rule of quadrants … ”) has also several consequences: since they contain the same numbers, they must have the same sum, product, parity, etc.. In the case of the “borders” of any puzzle, the opposite borders (left and right) must contain together exactly the same numbers as the top and bottom borders together (obviously, the corners are excluded for this purpose). And if you observe again the Graphic 1, the “wide” borders, left and right, top and bottom, have the same behaviour.
However, it’s a little early to talk about strategies, tips, etc., based on these curious properties.